Shell Theory is a field of physics, mathematics, architecture, topography and engineering which provides insight into masonry dome structures. Today I’ll be looking at Shell Theory in the context of many topics which I’ve already written about on this blog, and tying several things together toward some valuable insight into masonry dome and arch structures.
Shell Theory has its roots in the Euler–Bernoulli Beam Equation (also known as the “engineer’s beam theory”, “classical beam theory” or just “beam theory”) which was developed around 1750. This theory describes a means of calculating the deflection and load-bearing capacity of a beam. This theory was not fully utilized until the late nineteenth century, with the construction of both the Eiffel Tower and the Ferris Wheel. This theory played a major role in the engineering developments of the Industrial Revolution.
The Euler-Bernoulli equation describes the relationship between applied load and deformation, where E is the elastic modulus; I is the second moment of area; w is the deflection of the beam at some point, x; and q is the distributed load. Don’t be scared by this equation! It says if you push a beam this hard, in that place, it will bend this much, that’s all.
Plate Theory is an expansion of beam theory to thin-walled structures, or “plates.” The plate is assumed to be thin enough that it can be treated as a two-dimensional element, rather than as a thick beam, as in beam theory. Plates are assumed to be flat, or planar.
If the plates (in plate theory) are curved in two dimensions, then we enter the realm of shell theory. A round cylinder is curved in one dimension: whereas a sphere, or dome, is curved in two dimensions. A plate bent in two dimensions will have greater flexural rigidity than a plate bent in one dimension, and be more rigid still than a flat plate. (Flat plates are still shells; they’re just boring, weak, flat shells).
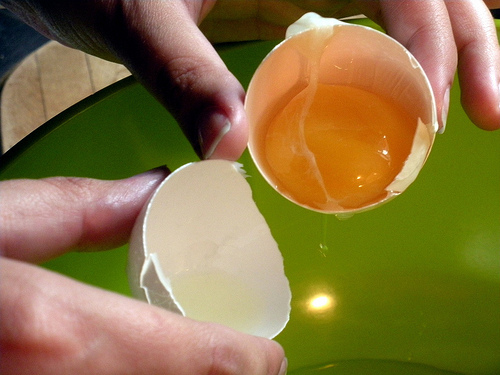
One good illustrative example of shell theory is provided by looking at an actual shell, like a chicken egg shell. If we return to Brunelleschi, who I talked briefly about here, it is interesting to note how he convinced his patrons (Medici) to allow him to build his famous dome. He simply took eggs and squashed their wide bottoms, so the thin tips were pointing up! Voila! He seemed to say, the egg creates a catenary arch which is simple, robust, rigid, symmetrical and will serve as a form to make the masonry dome, or duomo. His idea worked, it worked magnificently, and today we still have his duomo as a testament to his insight. It is interesting to note that Brunelleschi had a highly developed intuitive sense of shell theory centuries before this theory had been mathematically expressed and articulated by equations.
If we take this example of an actual egg shell and apply some of what was learned by Galileo’s mistake of applying his Square Cube Law to masonry structures (as I discussed here, here and here) the results are pretty astounding. The reader will recall that one critical fact of masonry arches is that they are scaleable. This means that if an arch’s span is doubled, it will remain stable so long as the wall thickness is also doubled. As long as proportions remain intact, a dome or arch remains stable, no matter how large it is made. This is a direct refutation of Galileo’s Square Cube Law. Galileo was wrong when he applied his law to masonry arches.
An actual chicken egg has a ratio of wall thickness to diameter of 7 to 1000. In other words, an egg 2 inches across has a shell which is 0.014 inches thick. If we “scale up” the egg so that it has a diameter of, say, 25 feet in diameter, then the walls would be only 0.175 inches thick! This points to the inherent strength of a masonry arch which is doubly curved, or domed. Any engineer must include a safety factor. I am planning to make triangular block to build a 25 foot diameter dome with wall thickness of just 4 inches. If directly compared with an egg shell, this provides an adequate safety factor of almost 23 (0.175 x safety factor = 4; safety factor = 22.857). A typical safety factor is usually around 10. Thus a 25 foot diameter dome made with block 4 inches thick would have a very high safety factor. Of course block differ from eggshell, so the comparison is tricky; more on that later.
I’ll talk more about Shell Theory and masonry domes in my next entry. This is a fascinating topic.









The question I thing is what is the perfect geometry for a dome or arch in order the to eliminate tension in whole structure. The answer is in www.uni-str.com. You can optimize masonry arch and dome geometry online.
ReplyDeleteHello Osman, I looked at your website. You have made available a catenary calculator. Catenary is not always ideal (See "Catenary reconsidered" entry on this site, February 26, 2012). A very large number of different types of shapes are required to construct these catenary structures. Furthermore in an earthquake with sideways motion, the catenary thrust lines shift, and will not correspond to the catenary your program calculates; it is a whole new shape (again, see "Catenary reconsidered"). Of course tension is never eliminated: it is simply resolved at the base (foundation) with either a massive buttress or a tension ring, or both. I like your approach to catenary code, it might be useful for some.
Delete